The Trajectory Normalizing Factor
By: Josh Kunz
The long-range shooting community is a niche market of the firearms world, and that niche is full of myths and misunderstandings about a lot of aspects of long-range shooting. One of the most common is the misconception that just because a caliber is larger or a bullet is heavier, it must therefore “cut the wind” better. Here is a simple, effective, and nearly fool-proof method to get a fast answer to, “which has a better wind call?” that doesn’t involve running ballistics through a calculator.
Many folks want a way to figure out what “the best” combination might be for a given task. This need is many times solved by doing detailed ballistics calculations. In today’s world of handheld computers (smartphones) this approach is much less onerous than in years passed but even today it still takes a lot more time to run a set of ballistic simulations than it does to solve a simple math problem on a 4 function calculator.
The Trajectory Normalizing Factor is a seemingly simple approach developed by myself to perform this exact task quickly and effortlessly when discussing caliber options with customers and friends for rifle building needs.
There are 2 basic inputs that are required and we can ignore a lot of the others that we need for the ballistic calculators. We are going to talk about comparing 2 calibers under identical conditions. Always. It’s not useful to compare combination A at sea level and combination B at the top of a mountain on a hot day. We need to simply know which is better under the same conditions because as those conditions change they change for both options.
Some basic rules and assumptions: Atmospherics are assumed identical.
Drop is important but for trajectory comparisons, it is secondary to the wind. Ignore drop concerns and worry about the wind.
The G-standard for the ballistic coefficients must be the same. The units on the muzzle velocity must be the same.
This means if Combo A uses feet/sec then Combo B must use feet/sec as well and if Combo B uses G7 standard, then Combo A must use G7 standard. Likewise, if Combo A only has data for G1 standard, then Combo B must use G1 or take the G7 value and convert it to G1.
Otherwise, the calculation is skewed.
OK, simple enough. What is TNF?
??? = ?? ∗ ??
Truly that simple.
Sample Calculation:
Combo A: Berger Hybrid 6mm 105gr flying at 3,030 fps (0.275 G7) Combo B: Hornady ELD-m 6.5mm 140gr flying at 2,740 fps (0.326 G7)
Which has a better trajectory?
??? =3,030???∗0.275=833.25 ?
??? = 2,740??? ∗ 0.326 = 893.24 ?
Based on the TNF calculated for each the 6.5mm option has a better trajectory. Below is the 1,000yd solution calculated for 0 DA showing the elevation & wind calls.
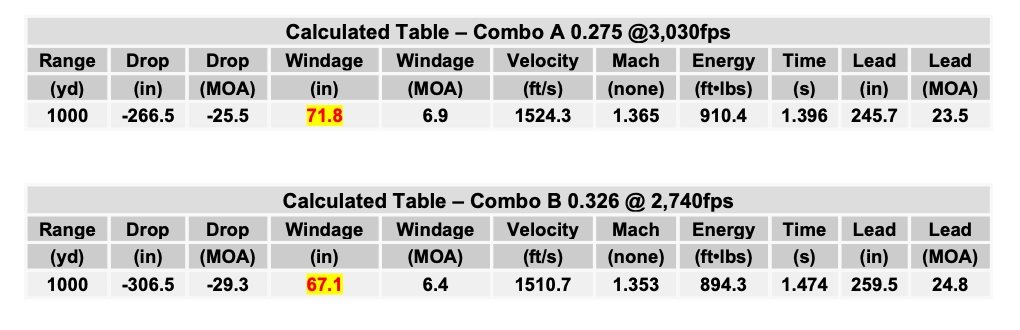
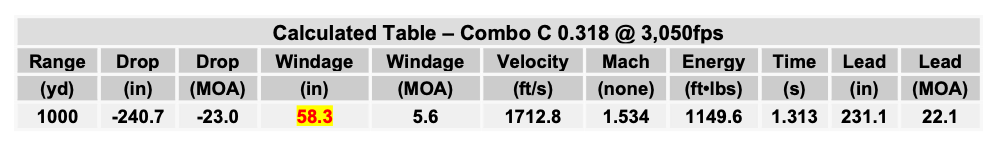
In this case the larger, heavier bullet going slower did better than the smaller faster bullet. But let’s look add in a 3rd case where that wouldn’t be true. Combo C is using a 100gr 6mm bullet with a very high BC. Lighter, faster, and better trajectory.
??? =3,050???∗0.318=969.9 ?
Let’s take it one step further by asking “If I have Combo A, how fast do I need to shoot Combo B to have an equivalent trajectory?” This is based on the age-old comparison of 2 bullets in the same rifle. Should we use the 180gr or the 162gr bullet because the 180 has more BC but the 162 can be shot a lot faster? Put more directly, if we have Combo A already figured out how fast does Combo B needs to go for it to be equivalent to Combo A?
Let’s take a look at the author’s 7mm SAUM shooting a Hornady 180 ELD-m at 2,870fps and figure out how fast the 162 needs to be shot to equate the wind call for the 180 at 1000yd?

Soon this concept will be built into a calculator will be available for free on www.patriotvalleyarms.com
By: Josh Kunz
The long-range shooting community is a niche market of the firearms world, and that niche is full of myths and misunderstandings about a lot of aspects of long-range shooting. One of the most common is the misconception that just because a caliber is larger or a bullet is heavier, it must therefore “cut the wind” better. Here is a simple, effective, and nearly fool-proof method to get a fast answer to, “which has a better wind call?” that doesn’t involve running ballistics through a calculator.
Many folks want a way to figure out what “the best” combination might be for a given task. This need is many times solved by doing detailed ballistics calculations. In today’s world of handheld computers (smartphones) this approach is much less onerous than in years passed but even today it still takes a lot more time to run a set of ballistic simulations than it does to solve a simple math problem on a 4 function calculator.
The Trajectory Normalizing Factor is a seemingly simple approach developed by myself to perform this exact task quickly and effortlessly when discussing caliber options with customers and friends for rifle building needs.
There are 2 basic inputs that are required and we can ignore a lot of the others that we need for the ballistic calculators. We are going to talk about comparing 2 calibers under identical conditions. Always. It’s not useful to compare combination A at sea level and combination B at the top of a mountain on a hot day. We need to simply know which is better under the same conditions because as those conditions change they change for both options.
Some basic rules and assumptions: Atmospherics are assumed identical.
Drop is important but for trajectory comparisons, it is secondary to the wind. Ignore drop concerns and worry about the wind.
The G-standard for the ballistic coefficients must be the same. The units on the muzzle velocity must be the same.
This means if Combo A uses feet/sec then Combo B must use feet/sec as well and if Combo B uses G7 standard, then Combo A must use G7 standard. Likewise, if Combo A only has data for G1 standard, then Combo B must use G1 or take the G7 value and convert it to G1.
Otherwise, the calculation is skewed.
OK, simple enough. What is TNF?
??? = ?? ∗ ??
Truly that simple.
Sample Calculation:
Combo A: Berger Hybrid 6mm 105gr flying at 3,030 fps (0.275 G7) Combo B: Hornady ELD-m 6.5mm 140gr flying at 2,740 fps (0.326 G7)
Which has a better trajectory?
??? =3,030???∗0.275=833.25 ?
??? = 2,740??? ∗ 0.326 = 893.24 ?
Based on the TNF calculated for each the 6.5mm option has a better trajectory. Below is the 1,000yd solution calculated for 0 DA showing the elevation & wind calls.
In this case the larger, heavier bullet going slower did better than the smaller faster bullet. But let’s look add in a 3rd case where that wouldn’t be true. Combo C is using a 100gr 6mm bullet with a very high BC. Lighter, faster, and better trajectory.
??? =3,050???∗0.318=969.9 ?
Let’s take it one step further by asking “If I have Combo A, how fast do I need to shoot Combo B to have an equivalent trajectory?” This is based on the age-old comparison of 2 bullets in the same rifle. Should we use the 180gr or the 162gr bullet because the 180 has more BC but the 162 can be shot a lot faster? Put more directly, if we have Combo A already figured out how fast does Combo B needs to go for it to be equivalent to Combo A?
Let’s take a look at the author’s 7mm SAUM shooting a Hornady 180 ELD-m at 2,870fps and figure out how fast the 162 needs to be shot to equate the wind call for the 180 at 1000yd?
Soon this concept will be built into a calculator will be available for free on www.patriotvalleyarms.com


