Introduction
Mr A. Mallock ("Vibrations of Rifle Barrels", Proceedings of the Royal Society, Vol. 68, page 327, 1901) may not have been the first person to put forward the proposition that a rifle can be "tuned" to generate "positive compensation" at a given range, but it shows that this idea has been around for well over a century and is not a new one.
The proposition for positive compensation is based on the fact that when any given batch or type of ammunition is chronographed, there is a always a spread in muzzle velocity observed about a mean. As a consequence, there will be a vertical dispersion in the fall of shot at the target, as the slower bullets in the sample take longer to travel down the range and so drop further than the faster bullets. It is easy to compute what the vertical dispersion should be for a given batch of ammunition due to the observed spread in muzzle velocity.
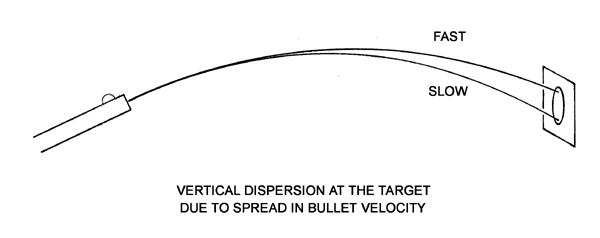
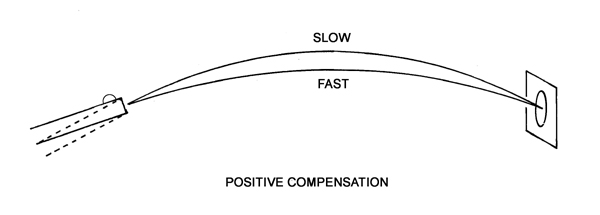
Curiously though, it is also often - if not usually - observed that the calculated vertical dispersion is not evident on the target. To explain this observation, the concept of "positive compensation" is invoked. It is generally recognised that the shock of the recoil forces in the rifle generate transverse vibrations in the barrel. For positive compensation, it is envisaged that the bullet is exiting the muzzle during an upward swing in the vibration at the muzzle, such that faster bullets (which arrive at the muzzle slightly earlier than slow bullets) are launched at a slightly lower angle into their trajectory than slower bullets. This will tend to reduce the
vertical dispersion in the group at the target resulting from the variation in muzzle velocity. And if the upward swing in the muzzle is exactly right, there will be complete positive compensation as the trajectories of bullets across the entire spread of muzzle velocities all meet at the same height on the target at a given range.
If there is (almost) general agreement on the principle, the many attempts since Mallock's paper to be more quantitative about the actual vibrations on a rifle barrel, and how they may be controlled to produce positive compensation, have left us little further forward than we were a century ago. Some attacks of note are summarised below.
The earliest attempt of note to measure vibrations on a rifle barrel (a Model 71 Mauser) was that of Cranz and Koch (�Untersuchungen uber die Vibration des Gewehrlaufs.� Abhandlungen d. math-phys. C1 D. Akedemie Der Wissenachaften, Vol. 69, page 747-775, Munchen, 1899.) who used high-speed photography to follow the motion of a wire attached to various parts of the barrel.
In 1912, Francis Carnegie ("The Vibration of Rifle-Barrels", Min. of Proc. of Inst. of Civ. Eng., Vol. 191, page 217-255, 1912-13 Pt. 1) built on the work of earlier experimenters (cited therein) in using a camera focused on a back-lit pin-hole which had been drilled in a thin sheet of metal attached to the barrel of the rifle. The vertical excursions of the barrel were thus recorded as the rifle recoiled to give the time-base on the photographic plate. Their test subject was the S.M.L.E. .303 service rifle, both stocked and de-stocked. They achieved some good results - notwithstanding the variable nature of the time-base of data obtained in this way, and that the fact that the barrel did vibrate before the bullet exited the barrel was firmly established.
Following on from Carnegie's work, a very determined effort was made to study the dynamics of a rifle under recoil at Woolwich Arsenal during the early 1920s. This work was finally published as an internal report. ("On Rifle Barrel Vibrations and their Effect on the Angle of Jump", R.D. Report No. 63, 1925) They also used the S.M.L.E. rifle as their test subject and showed how careful experimental methods can yield very useful results even when using comparatively primitive methods. In fact, it would be a long time before their results were bettered. This report was only de-classified in 1959.
In 1927, Captain Philip P. Quale used an oscillograph* for his attempt to measure the vibrations on the barrel of a 1903 Springfield ("The Vibrations of Rifle Barrels", American Rifleman, January 1, page 17-32, 1927).
(*An immediate predecessor to the cathode ray oscilloscope, but using a high speed drum camera to record the movements of a beam of light bouncing off a small mirror, which was attached to a coil suspended between two powerful magnets.)
A decade later, A.E. Martin and J. Muir ("Vibrations of a Rifle Barrel", Journal of the Royal Technical College Glasgow, Vol. 4, Part 2, page 213-239, 1938) used a cathode ray oscilloscope (a novel instrument in 1938) to measure the excursions of a phonograph needle, placed on the muzzle of a Lee-Enfield .303 rifle. They compared their results to the resonant frequencies of transverse waves on a uniform steel bar of similar dimensions to the rifle barrel, with results that were suggestive at best. The dynamic behaviour of a rifle barrel responding to a complex, impulsive, bending moment at one end, was (and is) not tractable in an analytic form and the computing power to tackle the problem numerically would not be available for another thirty years.
Harold Vaughn ("Rifle Accuracy Facts", Pub. Precision Shooting Inc. Connecticut, 1998) took another tilt at the problem. For his experimental work, Vaughn used an accelerometer on the muzzle of the barrel. The resulting trace of acceleration with time had to be integrated - once to obtain the vertical velocity of the muzzle, and again to get at the position with time. These results were of limited use by themselves. The main value of Vaughn's experimental results was to check his theoretical computer model, which used a numerical "lumped parameter" approach to model the rifle barrel vibrations. The results were more convincing than those of Martin and Muir, sixty years before, but it was clear that Vaughn's computer model was not reproducing the dynamics of the rifle barrel with sufficient accuracy to be useful in the problem of achieving positive compensation by design.
The increasingly powerful computers of modern times has enabled various interested individuals to run highly sophisticated Finite Element Analysis (FEA) computer simulations of rifle barrel dynamics as the bullet is travelling up the barrel. Probably the most sophisticated computer simulation to date is that run by
Al Harral using the LS-Dyna FEA code. This code was developed at Lawrence Livermore National Laboratories, where Al Harral worked using the LS-Dyna code to analyse mechanical structures. Al Harral realised the potential of harnessing the muzzle vibrations to achieve positive compensation at a given range and has attempted to design barrels specifically contoured to give the desired result. His work has lacked the experimental confirmation needed to verify his computer modelling, however.
Also notable amongst these campaigns using FEA modelling of rifle barrels is that of Kevin N. Schwinkendorf and Steven P. Roblyer ("Simulation of the Vibrational Response of a Rifle Barrel During Firing", Proceedings of the Advanced Simulation Technologies Conference, organized by the Society for Computer Simulation, page 66, April 5-9, 1998). It was the belief of these two authors that if the muzzle vibration could be "tuned", so that if the rate of change of muzzle angle was minimised (zero) at the time of bullet exit, the vertical dispersion of the group at the target due to the variation in muzzle velocity would be minimised. This assumption is only justified if the launch time of the bullet with reference to the vibration cycling on the barrel does not change with muzzle velocity. They ran simulations for various muzzle weights to tune the vibrations for a null at the bullet exit time. Practical tests were to follow to verify the simulation, but to my knowledge no results of any such tests have been published.
The influential American rimfire gunsmith Bill Calfee, in an article written for Precision Shooting Magazine ("I'm Feeling Those Good Vibrations AGAIN!" Vol. 52, No. 11, March 2005) presented a rather novel view on how barrels vibrate, and also expressed his belief that for best accuracy, barrels should be tuned so that the muzzle is "stopped" and there is no change in muzzle angle, or position, as the bullet is launched. It should be noted that Calfee's theories have absolutely no basis in fact and are mathematically untenable. But that does not stop it being the most quoted work in the popular press on barrel vibrations and the tuning of barrels.
Despite the increasing sophistication of the attack on the theoretical modelling of rifle barrel vibrations in recent years, experimental work of comparable accuracy to check the computer models has been notable in its absence. The work presented here is an attempt to start redressing the balance between experiment and theory so that future theoretical work can be better informed.
The apparatus
It is clear that the variable that is required to be measured is the angle of the muzzle of the barrel to the horizontal, and the way this varies with time as the bullet exits the barrel. All the experimental attempts listed above have measured the position, velocity, or acceleration of a point on the rifle barrel. The best these results could hope to do was inform a theoretical model which could calculate the rate of change of angle at the muzzle, but which could also generate data on the predicted position, velocity and acceleration at any point on the barrel with time with which the experimental results could be compared. It would be helpful to have experimental results that could stand alone as a useful tool in determining the optimum vibrations for positive compensation, rather than be a test of a theoretical model which would be depended on to generate the useful information.
Using a mirror attached to the muzzle in some way, so as to reflect a beam of light, is an obvious route. The excursions of the reflected beam would be a direct function of the vibrations of the barrel at the mirror, and could be measured in a number of ways. However, such an apparatus would be difficult to set up and very cumbersome, as its sensitivity would proportional to its size and the angular variation expected is small.
The method finally chosen was to attach a thin sheet of polarising plastic to the muzzle. Beams of light would be shone through the polarising sheet onto polarised detectors. If the planes of polarisation of the polarising plastic sheet and the polarised detectors were set at 45 degrees, the change in signal in the detectors would be proportional to the change in angle of the polarising sheet - and so the muzzle of the barrel. By using two detectors polarised at 90 degrees to each other and combining the two signals in a differential amplifier, the signal would also be insensitive to any common-mode changes such as in the intensity of the light sources.
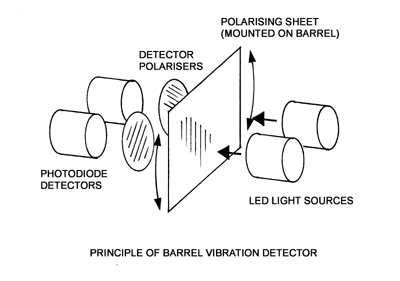
Such an apparatus could be compact, sensitive, easy to set up, and would have the added benefit that it would only be sensitive to changes in angle of the polarising plastic sheet about an axis perpendicular to the plane of the sheet. It would not be sensitive to any other degree of freedom such as a change in position either up-down, backwards-forwards or sideways or rotation about any other orthogonal axis.
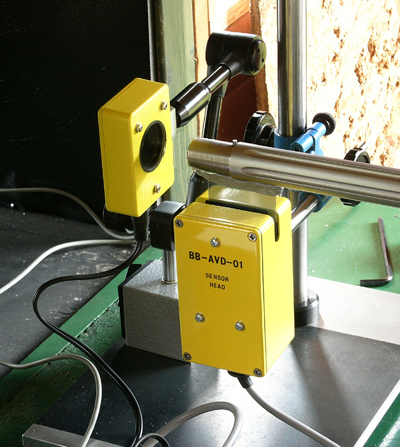
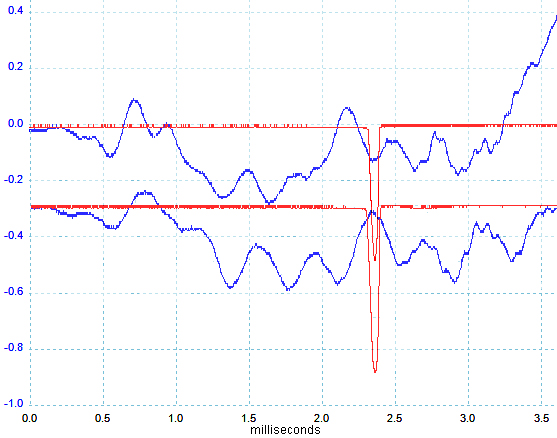
A "muzzle gate" was also required to detect the exact moment the bullet exited the barrel. This was a simple photoelectric device, using photodiodes to detect the interruption of light from an LED array as the bullet passed between them. The experimental setup is shown above. The polarising sheet is attached temporarily to the bottom of the barrel using "Blu-Tac", a malleable plastic adhesive. The bottom of the sheet is seen sitting in the slot of the sensor head, which contains the light sources, the polarised detectors and the first stages of the differential amplifier. The muzzle gate is seen sitting in front of the muzzle of the barrel. A contact microphone is attached to the action using a magnet and this detects the sound of the firing pin hitting the case. This signal is used to trigger the digital oscilloscope used to record the signals from the sensor head and the muzzle gate.
Variation of muzzle velocity with bullet exit time
The first step in deducing what rate of change of angle at the muzzle is required to give positive compensation, is to know how the exit time of the bullet at the muzzle varies with muzzle velocity.
For this experiment, the muzzle velocity was determined using an Ohler 35 chronograph. The two sky screens were separated by four feet and they were centred on a point five feet ahead of the muzzle. The absolute value of the muzzle velocity was not too critical for this experiment as it was the differences in muzzle velocity that were of interest. Because of this, it was important that the 'jitter' on the measured muzzle velocity was minimised. In an attempt to minimise the jitter of the chronograph, an LED array forming a 'light bar' 6 inches long and of width 0.08", was placed 8 inches above each sky screen. This formed a thin light source above each sky screen of uniform and constant brightness along its length.
To measure the variation in bullet exit time from the muzzle, it would seem obvious to measure the variation in the interval between the contact microphone trigger signal (ie when the firing pin struck the case) and the bullet passing through the muzzle gate. But it was expected that there would be some variation in the initiation in burning of the primer and powder after the fall of the firing pin. This variation could mask the looked for variation in bullet exit time, due to variation in muzzle velocity, to some significant extent. However, it is the pressures generated by the burning primer and powder that gives rise to the barrel vibrations. While variations in the pressures may alter the amplitude of the vibrations, it was considered that the time history of the barrel vibrations would remain constant as this would be due to the mechanical structure of the rifle itself, and this would not change from shot to shot. The best way to measure the variation in bullet exit time would be with reference to the barrel vibrations, rather than the trigger signal.
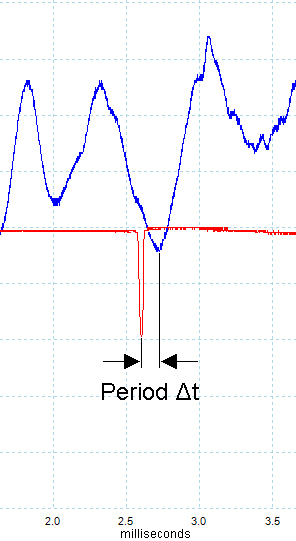
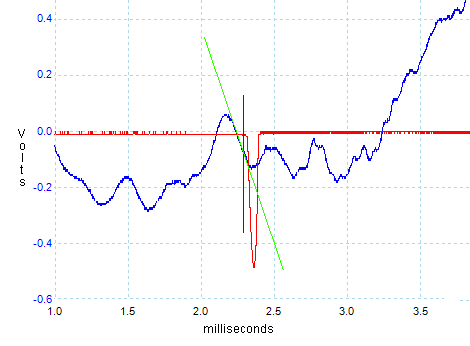
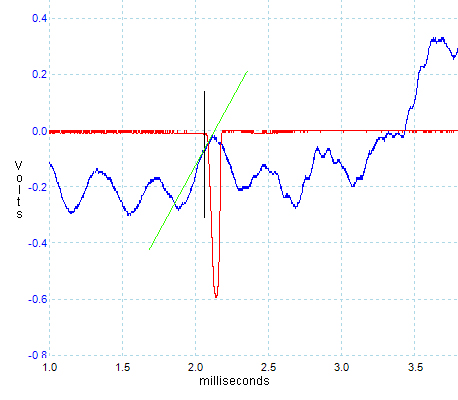
The trace on the right shows a portion of signal indicating the changing muzzle angle (blue) around the time when the bullet exited the muzzle, as indicated by the momentary downward 'blip' in the muzzle gate signal (red). The period 'delta t' from the muzzle gate signal to the following null in vibration signal was measured for each shot fired, along with the measured velocity.
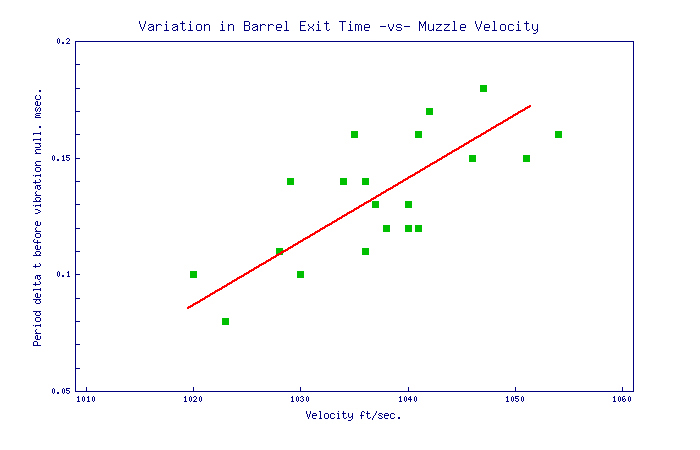
The graph below shows the results for Eley EPS Tenex ammunition. The data are rather noisy, but there is a fairly clear trend that for a change in muzzle velocity of 375 ft/sec., the exit time is changing by 1 millisecond. The barrel in this case was 26 inches long. This is expected to be a fairly universal constant applicable to all rimfire barrels of this length, shooting subsonic target ammunition.
Indeed, it may be that this is a universal constant for rimfire barrels of any length over six inches (approximately), after which friction is increasingly the dominant force on the bullet during its travel up the barrel. Thus, it can be argued that to first order, the spread in velocity of the bullets in the barrel will increase linearly with distance travelled up the barrel past the six inch point, and the rate of change of barrel travel time with velocity will remain fairly constant with length.
Variation in launch angle with muzzle velocity required for complete positive compensation at 50 metres
Using the
Trajectory program, it is trivial matter to compute the change in muzzle angle required for complete positive compensation at 50 metres, for a given range of muzzle velocity. So, for a velocity of 1010 ft/sec. the launch angle needed is 9.3 MOA and for 1060 ft/sec. the required launch angle is 8.5 MOA to hit the target at the same height.
The rate at which the angle of the muzzle has to change with muzzle velocity, so all the shots hit the target at the same height and there is complete positive compensation, is 0.016 MOA for 1 ft/sec. change in muzzle velocity.
Rate of change of muzzle angle required for complete positive compensation at 50 metres
Multiplying together the
Variation of muzzle velocity with bullet exit time and the
Variation in launch angle with muzzle velocity required for complete positive compensation at 50 metres, the required rate of change of muzzle angle with time is 6.0 MOA per millisecond for a 26 inch barrel.